Ftan mpa mpa mpa mpatmax mpa save mpaproblem solve prob

(a) SFs = 0		Fs – 100 sin b = 0
tw = F A w s				6								sin b
								¥	10	6	= 0.240
								¥	10	6
		Fn – 100 cos b = 0								Fn = 100 cos 14.34º = 96.88 kN
A = 800		¥	10	-6	= 825.74 ¥ 10–6 m2
s = F n						¥
A w						¥

PROBLEM 7.23

7.23 The steel pipe AB has a 102 mm outer diameter and a 6 mm wall thickness. Knowing that arm CD is rigidly attached to the pipe, determine the principal stresses and the maximum shearing stress at point H.

I = 1 2J = 2.0927 m4 Force-couple system at center of tube in the plane containing points H and K.												y
Fx = 10 ¥ 103 N				H
	Mz = – (10 ¥ 103)(150 ¥ 10–3) = – 1500 N◊m													y
T = My = 2000 N◊m				z									H	K
c = ro = 51 ¥ 10–3 m
txy = Tc J = (		2000 51		¥	10		-3	)
			¥	10						H
Transverse Shear, For semicircle
A = p 2r2 y = 4 3pr
											y				x
											H
= 27.684 ¥ 10–6 m3

V = Fx = 10 ¥ 103 N

t = (2)(6 mm) = 12 mm = 12 ¥ 10–3 m

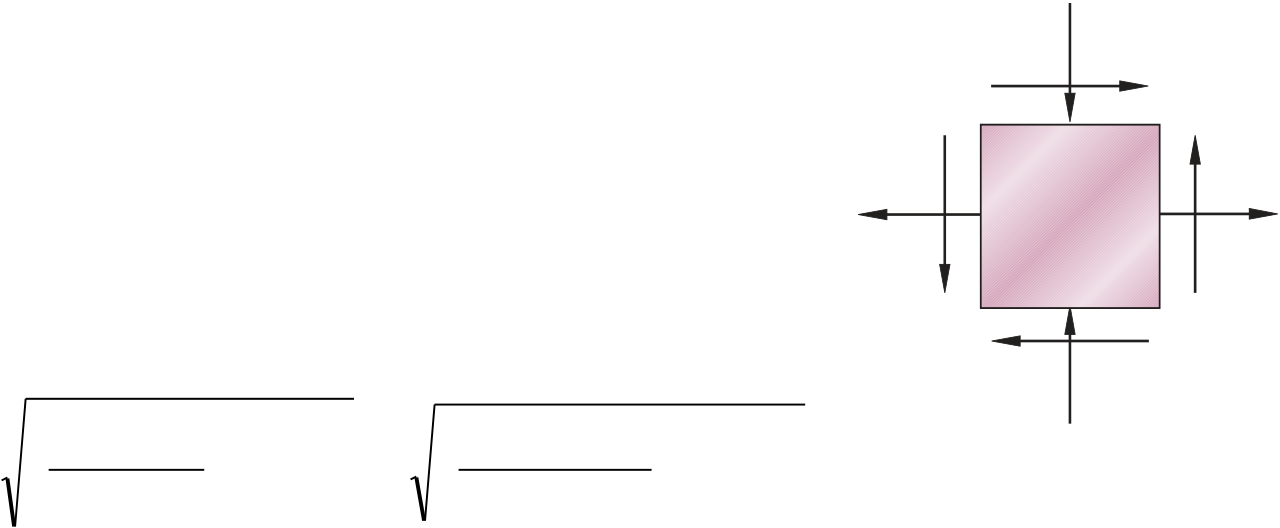
save = 1 2(sx + sy) = 0	R =	���	s	x	-	s	y	���	2	+	t
					2
smax = save + R = 35.39 ¥ 106 Pa = 35.4 MPa
smin =save – R = – 35.39 ¥ 106 Pa = – 35.4 MPa

Equivalent force-couple system at center of shaft in section at point H.

V = 1.8 kN M = (1.8 ¥ 103)(0.150) = 270 N◊m

	Chapter 7	829

PROBLEM 7.26

7.26 A mechanical uses a crowfoot wrench to loosen at bolt at E. Knowing that the mechanic applies a vertical 100 N force at A, determine the principal stresses and the maximum shearing stress at point Hlocated as shown on top of the 18 mm diameter shaft.

T = (100)(250) = 25000 N◊mm

sy = sx – 2U = 82 ± (2)(96) = – 110 MPa, 274 MPa

sx = sy + 2U = 75 ± (2)(30) = 135 MPa, 15 MPa

SOLUTION

qA = 1 2a = 52.97º

R = CG 2 + GX 2 = 10 2 + 35 2 = 36.4 MPa

tmax = R = 36.4 MPa

s¢ = save = – 50 MPa

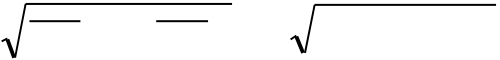
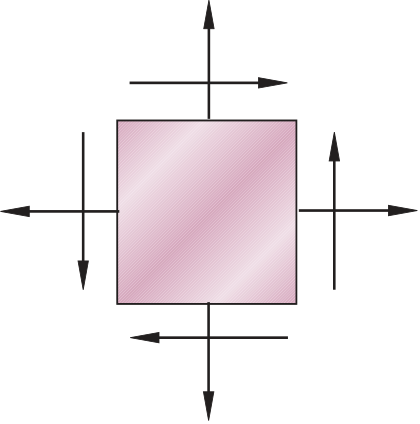
	sx = 120 MPa						txy = 60 MPa	t		(MPa)
	s= s	x	+	s	y
	ave		2						D

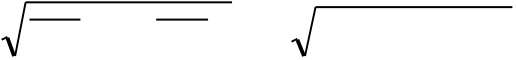
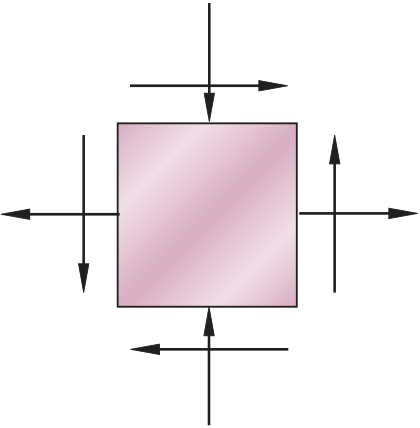
834												70 MPa
R = CF		2	+	FX	2	= 105	2	+	60	2
tmax = R = 120.9 MPa
s ¢ = save = 15 MPa
													10 MPa
7.34

stress, (b) the maximum in-plane shearing stress,
(c) the corresponding normal stress.

50 MPa

SOLUTION

been rotated through (a) 25º clockwise, (b) 10º
counterclockwise.

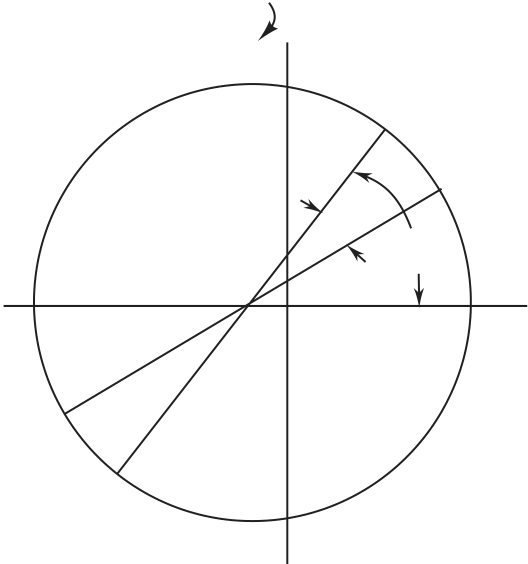
j = 50º – 30.65º = 19.35º

				Y		t
sy¢ = save – R cos j = – 86.6 MPa						C	X¢		s (MPa)
							X
							20°	j
j = 30.65 + 20º = 50.65º							30.65º
sx¢ = save + R cos j = 37.2 MPa							110 MPa
sy¢ = save – R cos j = – 62.2 MPa
	Solve Prob. 7.16, using Mohr’s circle.

wise.

SOLUTION

838														X�	t			Y	Y�s
2qP = 51.84º
R = FC				2	+	FX		2	= 55	2	+	70				51.84°
																		50°
= 89 MPa
															O		C	j
(a) q = 25º
																			MPa
j = 51.84º – 50º = 1.84º															X
sx¢ = save – R cos j = – 33.9 MPa

j = 51.84º + 20º = 71.84º

sx¢ =save – R cos j = 27.2 MPa tx¢y¢ = R sin j = 84.5 MPa
sy¢ = save + R cos j = 82.7 MPa.

7.17 and 7.18 The grain of a wooden member forms an angle of