The current moves the right the bottom the loop
Important Terms
alternatingcurrent
electric current that rapidly reverses its direction
electricgenerator
a device that uses electromagnetic induction to convert mechanical
energy into electrical energy
electromagneticinduction
inducing a voltage in a conductor by changing the magnetic
field around the conductor
induced current
the current produced by electromagnetic induction
induced emf
the voltage produced by electromagnetic induction
Faraday’slawofinduction
law which states that a voltage can be induced in a
conductor by changing the magnetic field around the conductor
Lenz’s law
the induced emf or current in a wire produces a magnetic flux which opposes the change in flux that produced it by electromagnetic induction
magnetic flux
the product of the magnetic field and the area through which the magnetic field lines pass.
| ε | = | − | N | ΔΦ | |
|---|---|---|---|---|---|
| V | S | = | N | ||
| V | P | N |
|
||
| I | S | = | N | ||
| I | P | N |
|
||
| P | = | IV | |||
In the 1830’s Faraday and Henry independently discovered that an electric current could be produced by moving a magnet through a coil of wire, or, equivalently, by moving a wire through a magnetic field. Generating a current this way is called electromagnetic induction.
If we move a rod perpendicular to a magnetic field, there is a magnetic force on the charges in the rod, sending the positive charges to one end of the wire and the negative charges to the other, as shown in your textbook. This polarization of charge creates a potential difference or emfε between the ends of the rod. If the rod closes a loop, the induced ε produces a current I around the closed loop.
B (out of the page)
The motional emf produced in the rod is
ε = vBL
| I | ε | vBL | |
|---|---|---|---|
| = | R |
Solution
Consider a small positive charge inside the rod. As the charge moves to the right with the rod, we can find the direction of the magnetic force on the charge by using right-hand rule no. 2.
B
Magnetic Flux and Faraday’s Law of Electromagnetic Induction, and Lenz’s Law
Consider a rectangular loop of wire of height L and width x which sits in a region of magnetic field of length 3x. The magnetic field is directed into the page, as shown below:
 |
 |
 |
 |
 |
|---|
The loop is in the plane of the page so that its area is perpendicular to the magnetic field
As the loop sits a rest in the magnetic field, the magnetic flux through it is constant, and is equal to BA = BLx. If the loop were tilted forward (toward you) at an angle φ relative
to the magnetic field, the flux would be
| Φ | = | BA |
|---|
L
 |
 |
 |
 |
 |
v | |
|---|---|---|---|---|---|---|
 |
 |
 |
||||
 |
 |
 |
Lenz’s law states that the induced emf or current in a wire produces a change in flux which opposes the change in flux that produced it.
The negative sign on the equation for the induced emf above tells us that the new change in flux works against the old change in flux. This is essentially a statement of
conservation of energy.
| − | ΔΦ | − | Δ | ( BA | ) | |||
|---|---|---|---|---|---|---|---|---|
| ε | = | = |
|
|||||
where A is the area of magnetic field which is enclosed by the loop of wire. In the position of the loop shown, this area would be Lx. So, the equation for ε becomes
Faraday’s law of induction is illustrated in the excellent examples in your textbook, and in the review questions and free response problem that follow.
The Electric Generator
| N | S |
|---|
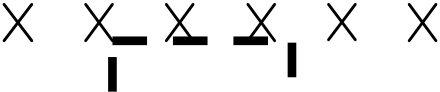
1. All of the following can induce and
emf in a coil of wire EXCEPT 3. A generator(A) moving a magnet through the coil (B) placing a stationary coil of wire in an increasing magnetic field
(C) placing a stationary coil of wire in a stationary magnetic field.
an decreasing magnetic field (D)converts electrical energy into heat
(E) moving both the coil of wire and a energy.
magnet pulled back out of the coil in the
opposite direction as it went into the 4. Which of the following is implied by
(B) There will be a current produced in the coil in the opposite direction as
the current that produced it.
(D) The current produced must be stronger than before.
(E) The current produced must be weaker than before.
L
271
Free Response Question
Directions: Show all work in working the following question. The question is worth 15 points, and the suggested time for answering the question is about 15 minutes. The parts within a question may not have equal weight.
| a |
|---|
B
(b) Is the direction of the induced current in the loop clockwise or counterclockwise? Briefly explain how you arrived at your answer.
When the loop enters the magnetic field, it falls through with a constant velocity. (c) Calculate the magnetic force necessary to keep the loop falling at a constant velocity. (d) What is the magnitude of the magnetic field B necessary to keep the loop falling at a constant velocity?
2. B
Pushing the north end of a magnet into a coil will produce a current in the opposite direction than if the north end were pulled out of the coil. Opposite magnet velocities will create opposite currents.3. A
Mechanical energy is put into a generator, and electrical energy is produced in the form of current.
| P | = | I | 2 | R | = | ( .0 012 | A | Ω | ) | = | .1 44 | x 10 | − | 3 |
|---|
(c) 3 points
| FB | = | IaB | = | |||||||||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
|
||||||||||||||||||||||||
| B | = | F B |
|
|||||||||||||||||||||
| = | BLv | |||||||||||||||||||||||
| B | F B | R | = | ( 15 | N | )( 0.5 | Ω | ) | s | ) | = | 10 | 2. |
|
||||||||||
| a | 2 | v | ( 4.0 | m |
|
m | / | |||||||||||||||||



