Exponentials and Logarithms Homework Help

Math - Exponentials and Logarithms

A logarithmic function is, putting it simply, another way of expressing an exponent. It is a function that says, "give me a number, and I will tell you how many times I need to divide by another number (called the base) before I get to 1.

" A more mathematical way of expressing the function is:

Log_b (x) = y

if and only if

b^y = x

The reason b is called the base is because it is the number that is being successively multiplied (when we are dealing with exponents) or divided (when we are dealing with logarithms). x in this case is the number that a specific b ^y evaluates to, and y is the exponent that you raise b to in order to get x.

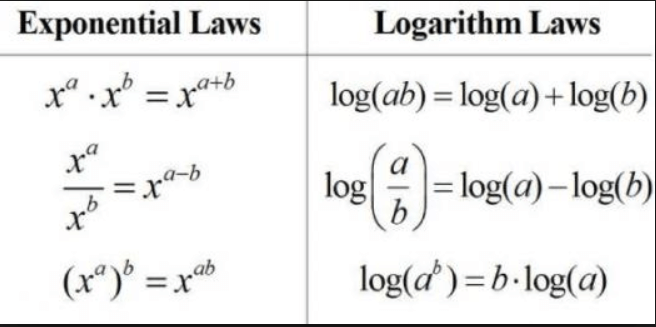
When I first learned logarithms, I found it confusing that there were 3 variables floating around, so let’s look at an example and evaluate y:

y = log_8 (64)

I'm going to demonstrate two ways of thinking about and solving logarithms. One way is to use successive division and count the number of times we need to divide until we reach 1. We always divide the thing we are trying to evaluate (the number in the parentheses) by the base.

64 / 8 = 8 (one division)
8 / 8 = 1 (two divisions)

So now we look at the number of divisions we performed, and that is our solution for y.

y = log_8 (64)
y = 2

Another way to solve logs is to re-write them according to the equivalence relations log_b (x) = y iff (if and only if) b^y = x.

y = log_8 (64)

in this equation, b = 8 (it’s the subscript that comes right after log notation) and x = 64. According to our equivalence relation (formula that says two things will always be equal) we can rewrite that in exponent notation.

8^y = 64

Now we have this in a form where we can use root notation to solve algebraically.

8 ^y = 64
8 ^y - 2) = 1

The only number that you can raise 8 to is 0 (recall any number to the power of 0 is 1).

y - 2 = 0
y = 2

This method also shows us why the successive division works. As long as your x is your base raised to some power (which it must be, although sometimes logarithms aren't exact) you will always be able to divide by your base enough times to get 1.