Factoring Polynomials Homework Help
Factoring Polynomials
Polynomials are relation between 2 variables. It is called polynomial when it has more than 2 terms.
Example y = ax^2+bx+c.
This is called 2nd degree polynomial where a,b,c are called coefficients. Degree of polynomial is known decided the maximum power in the equation
Example y= ax^3+bx^2+cx+d
It is 3rd order polynomial. And power must be positive integer for an equation to be polynomial.
Factorizing of polynomial means to find the roots of the equations or see where the graph of polynomial cut x axis. There are many ways to factorize polynomial, complexity of finding roots increase with power as someone said, {"With Great Power comes great effort to find Roots"}. Most basic method or way is hit and trial. Then for 2nd order equation we have D method and decomposition. For Cubic equation we have to find one root by hit and trial and later by Polynomial division
In another words:
Polynomials are expressions of two or more algebraic terms.
Example,
4x+2 is a polynomial (binomial specifically, with 'bi-' meaning 2 and '-nomial' meaning terms).
Factoring is the process by which we determine what was multiplied to get the given quantity.
For example,
you could factor 6 as (2)*(3).
Or, to use a simple polynomial example, 4x+2. You can factor a 2 from each of the terms (as though you were dividing it).
So 4x+2 factors out to (2)*(x+1)
A trickier polynomial to factor would be x^2 + 3x + 2.
To do this, look to see which 2 numbers can be multiplied by each other to get 2, and can be added to get 3. (The 2 is the constant and the 3 is the coefficient from 3x in the expression).
The only numbers which fit these rules are 1 and 2.
((1)*(2) = 2, (1)+(2) = 3).
Now write (x + ___) * (x + __)
Place the 2 and the 1 in the empty places -
Note that the order does not matter.
So x^2 + 3x + 2
factors out to
(x+1) * (x+2).
Example of Factoring
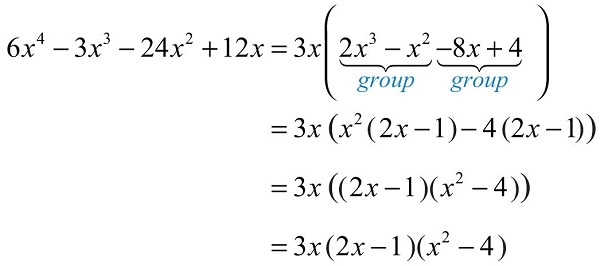
Math Tutorials
- Math - Polynomials
- Math - Series and Sequences
- Math - Dividing Polynomials
- Math - Factoring Polynomials
- Math - Solving Quadratics
- Math - Solving Inequalities
- Math - Solving Equations
- Math - Complex Numbers
- Math - Matrix
- Math - Inverse Functions
- Math - Graphing Quadratic Functions
- Math - Graphing Polynomial Functions
- Math - Functions
- Math - Exponentials and Logarithms
- Math - Cramer's Rule
- Math - Absolute Value & Inequalities

