Inverse Functions Homework Help
Math - Inverse Functions
If the function f is defined from the set X (domain) to the set Y (range), then there exists another function g from the set Y to the set X is an inverse function off if,
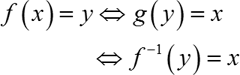
The inverse of any function is possible when unique value exists. In other words, if it is possible to have the same function value for different x values, then the inverse does not exist.
The graph of y = f (x) and y = f -1 (x) is shown in the below graph:
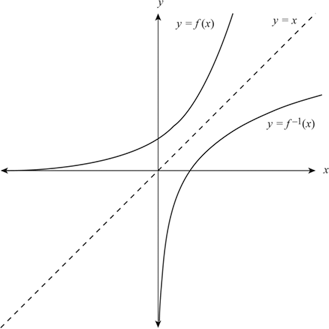
The graph of a function y = f (x) and its inverse y = f -1 (x) are mirror images of each other about y = x.
The inverse of any function can be obtained by using the steps given below:
Step 1: Fix a y for f (x).
Step 2: Change x and y.
Step 3: Obtain the value of y, say f -1 ( x).
In another way Inverse functions perform opposite operations on one another. That is, two functions f(x) and g( x) are inverse if and only if they are both one-to-one, meaning that there is only one output for each input, and if f composed ofg at x and g composed of f at x both return the value x:
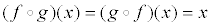
.
If f(x) and g(x) are inverse, then the derivative of g(x) is
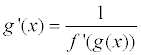
as long as
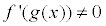
.
A function has an inverse if it is differentiable and its derivative is always non-negative or non-positive. Logarithmic and exponential functions are inverses of one another, such as exand ln x, as are the circular trigonometric functions and the inverse trigonometric functions, such as sine and arcsine.
An inverse is basically an opposite up has down, left has right, loud has soft, etc.
In arithmetic, some common inverses (or opposites) are adding and subtracting and multiplying and dividing. If we take a value of three and add four to it (3 + 4 = 7), the way to undo that addition is to subtract four, which gives us our three back (7 - 4 = 3).
Similarly, if we multiply that three by five (3 * 5 = 15), we can undo that multiplication by dividing by five to get back our original three (15 / 5 = 3). The key word here is "undo"; an inverse undoes what the original did. So how do we apply this to functions?...
Take the parabola f(x) = x2. To find its inverse, we must discover a function (called f-1 (x) or "f inverse of x") that, for every value of x we put into f(x), it will take that value f(x) and spit out x. Thus, f-1 (x) will undo anything that f(x) does! However, just like with arithmetic, this process shouldn't only go one way: anything that the inverse function f-1(x) does should be undone by our original function f(x).
If you've taken algebra, you should intuitively know that the simplest way to undo x2 is with a square root (sqrt(x)).
indeed, if f(x) = x2, f-1(x) = √(x).
So, in summary, an inverse function undoes whatever function it is an inverse of. This property doesn't just apply to simple functions like x^2 or 5x, it applies to functions like f(x) = 2cos (ex - 3) + 4x 3, the inverse is just a little more complicated.
Example 1 Inverse Functions
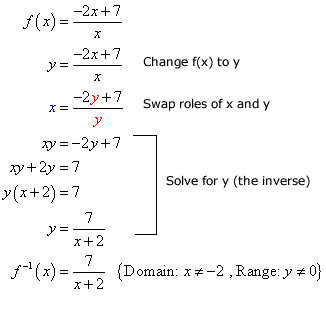
Example 2 Inverse Functions
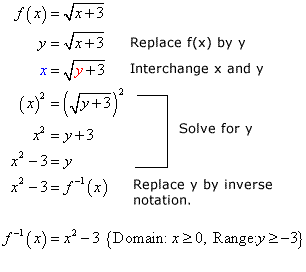
Math Tutorials
- Math - Polynomials
- Math - Series and Sequences
- Math - Dividing Polynomials
- Math - Factoring Polynomials
- Math - Solving Quadratics
- Math - Solving Inequalities
- Math - Solving Equations
- Math - Complex Numbers
- Math - Matrix
- Math - Inverse Functions
- Math - Graphing Quadratic Functions
- Math - Graphing Polynomial Functions
- Math - Functions
- Math - Exponentials and Logarithms
- Math - Cramer's Rule
- Math - Absolute Value & Inequalities

